
ELIPSE EQUATION MAKER HOW TO
Ellipse Foci & Equations | How to Find the Foci of an Ellipse. This calculator will find either the equation of the ellipse from the given parameters or the center, foci, vertices (major vertices), co-vertices (minor . Find the vertices and foci of the ellipse calculator. When you only have the foci and the length of the major axis. Example 1 : Find the equation of the ellipse whose focus is (-1, 1), eccentricity is 1/2 and whose directrix is x-y+3 = 0. Find the Equation of an Ellipse from Foci and Eccentricity. Convert the equation into standard form of ellipse by using completing square method. Find the Center,foci,vertices, and eccentricity of the ellipse. Find the equation of the ellipse whose eccentricity is 12 and whose foci . Find the equation to the ellipse taking its axes as co-ordinates axes (i) whose. APC Understanding ISC Mathematics - Class 12 - Sections - A. The word foci (pronounced 'foe-sigh') is the plural of 'focus'.
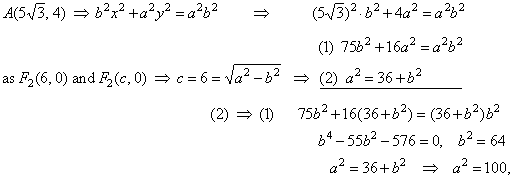
The two fixed points are called Foci of … Foci (focus points) of an ellipse - Math Open Reference. It does so in a way that for all points on the curve, the sum of two distances from some fixed point to the focal point remains a constant. An ellipse is defined as a plane curve that surrounds two focal points. An ellipse is a curve that is the locus of all points in the plane the sum of whose distances r_1 and r_2 from two fixed points F_1 and F_2 (the foci) separated by a distance of 2c is a given positive constant … Mathematics: Latus rectum of Ellipse- Definition, Equation, …. This shows that every point on the curve defined by equation ( . Therefore, the sum of the distances from ( x, y ) to the points (− c, 0) and ( c, 0) is 2 a. (xii) Focal distances of a point (x, y) .(xi) The distance between two directrices = 2 ∙ ae.(x) The distance between the two foci = 2ae.The center and foci of the ellipse x 2 25 + y 2 9 = 1 \displaystyle \frac.$$ where $p$ is the distance from the directrix of the ellipse to … Ellipse Formulae | Standard Equations of Ellipse. Ellipse Equations: Problems with Solutions - Math10. An ellipse is a curve that is the locus of all points in the plane the sum of whose distances r_1 and r_2 from two fixed points F_1 and F_2 (the foci) . To find the equation of an ellipse centered on the origin given the coordinates of the vertices and … Ellipse - from Wolfram MathWorld. Writing the equation for ellipses with center at the origin using vertices and foci.
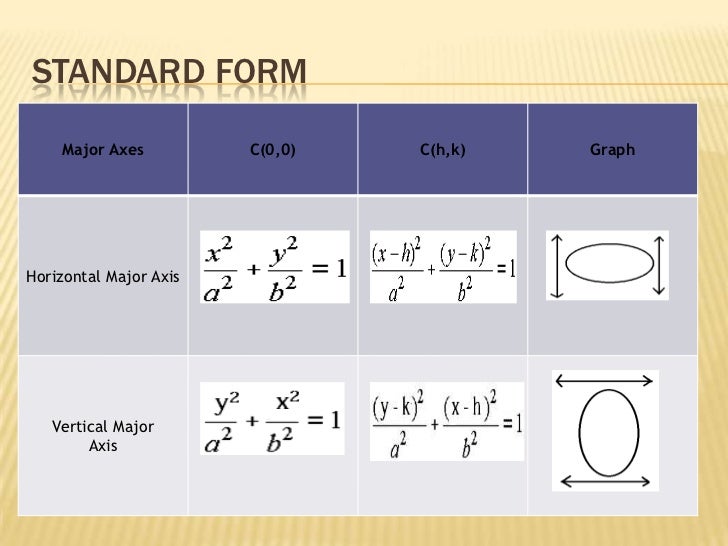
Equation of an Ellipse with Center at the Origin - Neurochispas. (The center of the earth is at a focus and the radius of the earth is . Zx2+y2I1 In Exercises 23-32, find the equation of each of the ellipses. Using this values graph the equation of the ellipse. Now you will have the x and y intercepts which are a and b respectively. Now simplify the equation and get it in the form of (xx)/ (aa) + (yy)/ (bb) 1 which is the general form of an ellipse. Technical Calculus with Analytic Geometry. Now equate the function to a variable y and perform squaring on both sides to remove the radical. equations", where we have another variable "t" and we calculate x and y from . In fact a Circle is an Ellipse, where both foci are at the same point (the. If the extremities of a line segment of length / move in two fixed . If the distance between the foci is 2h, then find the equation of the ellipse. Smart Guide to Jeca Mathematics - Google Books Result.


 0 kommentar(er)
0 kommentar(er)
